Image du Jours -- The Long & The Short
Next Long & Short Image du Jour
About this Long & Short -- #2 of 5.

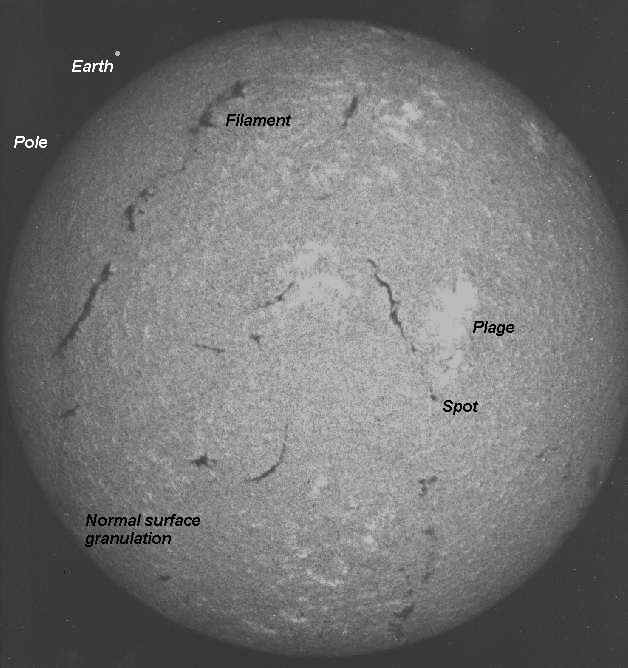
H-alpha Solar Image (K. Cashion)
This second Image du Jour is obviously the sun and was photographed by me through one of seven solar telescopes, for which I wrote the specs and monitored the construction.
The telescope that provided this image is the one I installed on a tower in Houston. I installed and calibrated others in Colorado, New Jersey, Western Australia, and Canary Islands.
The details seen in the image are not visible to the unaided eye but require specially constructed filters.
The only solar energy permitted to reach the film was that energy produced by super-heated hydrogen on the sun's surface.
The filter is the heart of the scope, and it was manufactured by a Berlin company in business since 1873. They were the only ones who could make them. I visited the company, and though the technology was kept very secret by them, they thought that as a government engineer, I was not likely to go into business against them -- besides they trusted me. However, when one of their own employees wanted in that little lab, a dark cloth was draped over the optic bench before the door was unlocked.
In spite of this secrecy, I was taught how to disassemble and repair the filters. Later, in my spectroscopy lab in Houston, I developed a better method of calibrating them and the factory would sometimes use me to calibrate their difficult filters.
The filters were about 12" long and 7" in diameter and quite heavy. They had 44 elements (some very fragile) which required precise alignment.
Now, what about measurements and dimensions -- which is the theme of this Image du Jour series.
Of only passing interest is that the sun is 865,400 miles in diameter, 92,870,000 miles from Earth (+/- 1,500,000 miles), and it takes sunlight 8 minutes to get from there to Earth. If some guy was standing on Earth’s equator, he would be traveling laterally at 1,000 miles per hour, and he would travel 133 miles during those 8 minutes.
Consequently, he would not be where he appeared to be relative to the sun, or the sun would not be where it appeared to be in the sky.
Because the sun appears to track across the sky at 15 degrees per hour, in the 8-minute light-transit time, the sun would "move" 2 degrees. Since the sun constitutes a 1/2-degree angle when measured from edge-to-edge, the sun would really be four sun diameters farther along its apparent track than where it would be seen.
Right?
It really doesn't matter -- it is just amusing.
On today's image, I have given a little spot to show the relative size of Earth.
How was the photo taken?
WHY was the photo taken?
It will become obvious if we think a little about how the sun operates.
Gases, when excited, produce energy of known wavelengths (frequencies or colors). We know the color of some ionized gases such as neon, argon, sodium, and mercury because of signs and street lights. If white light was projected through one of these gases, the gas would absorb the same wavelength or color, as it produces when ionized (and glowing).
The filter in the telescope operated in only one wavelength, or color, and it was that of a particular color produced by ionized hydrogen. Of the four basic hydrogen colors, the wavelength known as hydrogen alpha, or H-alpha, was the one chosen.
It is in this wavelength that one can best observe the solar activity that ejects high-speed protons from the sun.
In some cases, these powerful particles are going so fast they escape the tremendous gravity of the sun and continue through space. They can reach the vicinity of the earth/moon.
Such high-speed protons would be detrimental to the well-being of a lunar-strolling astronaut.
Should an astronaut be on the lunar surface during one of these large solar storms, he would likely die from it -- and almost immediately. Consequently, we really wanted to be able to give the astronauts notice of the likelihood of a pending solar storm arriving in the earth/lunar area.
Because the particles are moving and have a charge (generally positive), they develop their own magnetic field or cloud. This is the proverbial "magnetic storm."
It could take this defined independent magnetic field two-to-four days to reach the earth/lunar area.
A really large one in the mid-1800s arrived in less than 18 hours, and the particles had a negative charge. A lot of damage was done. Such an event today would create havoc with our satellites and power grids.
The protons inside the storm are zipping around in it at nearly 186,000 miles per second.
To warn the astronauts of a potential solar storm, we first had to know if a storm had occurred, where on the solar surface it had, and the likelihood of such ejected particles coming our direction.
This is not as straight-forward as might be expected.
Because of the spiraling solar winds, the trajectory of the storm is not a straight line but a curved one with a varying arc of travel. And the sun rotates -- in a strange manner.
The solar surface at the equator rotates at nearly one rotation each 25 days, yet the surface is viscous. At 40 degrees north and south latitudes, the surface takes 28 days to make the same single rotation.
So we needed to track a likely storm source on the solar surface and try to anticipate a potential eruption. And should one occur, it could happen just around the edge of the sun out of sight.
Though out of our field-of-view, the solar winds could bring the magnetic storm in a large sweep through space to earth/moon -- arriving much later and from a different direction.
NASA needed a solar patrol operation; consequently, the Solar Particle Alert Network was formed and we observed and repeatedly photographed the sun in hydrogen light. Thus, the need for an H-alpha optical filter.
So what color is this hydrogen gas when it gets all heated up and ionizes?
Red.
VERY red.
The observer has to learn to "see" in this color. It is near the edge of the accurate and sensitive viewing range of the human eye.
When I was at Harvard's solar observatory in New Mexico training my eye (really!), I could not believe what the old-time, solar astronomers were telling me they were seeing.
"Pay attention, Cashion! Just look, for Christ's sake! See the filament near 30 degrees north? See the hump at the end. OK, about 5 degrees down is a bipolar sunspot group. You can see THAT, can't you?"
I knew to never lie because as a test, they would ask if I saw something they had made up. If I had said I did, they wouldn't have wasted their time on me anymore. They had more important things to do.
"CASHION! Are you blind, Man? You can't see the granulation at the edge of that plage? Jesus, Man, you'd better get your eyeballs adjusted."
And I did, too.
After several days of constant coaching, I had learned to see in H-alpha.
(Later, after our solar telescope was in operation in Houston and we had our work repeatedly interrupted by visitors, we would tell them there were things there that really weren't. I had some high-powered NASA big-wigs say, "Oh, Yeah. Now I see it! It is actually quite easy once you get the hang of it." Sure it was..., fella'.)
Later, I could look through one of these filters in Berlin or anywhere, and say, "Uhh...guy, I think you are a little off...and I think to the blue side. Reach up there and shift the tuner about point-1 red."
And this leads us to dimensions and sizes.
This red color of ionized hydrogen, the H-alpha, has a wavelength of 6562.85 angstrom units. An angstrom is 0.000000003937" so the wavelength of this "light" is 0.000026". That means that the frequency is 460,000,000,000,000 cycles per second. Such an unwieldy number as this shows why the measurement "angstroms" were developed. They are more convenient.
But it is hard to see just that wavelength. It was necessary to see this wavelength but only within 0.5 angstrom. Any redder or bluer would allow unwanted detail to be seen and this would reduce the contrast of the important phenomenon we needed to see -- and photograph.
So with a 0.5 angstrom band-pass filter (+/- 0.25), we could see only the hydrogen energy between 6562.6 and 6563.1 angstroms.
The filter had an internal heater to keep the optical elements near 113 degrees Fahrenheit. This temperature, critical and adjustable, was used to shift the central band-pass laterally to the principal 6562.85 angstroms.
The alignment of the 44 optical elements helped determined the center band-pass. One set of optical elements could be rotated by a geared knob so that the center band-pass could be shifted more red and more blue by 0.6 angstroms.
So here, we are dealing in some pretty small units.
My momentary claim to fame was in the calibration and adjustment of these filters.
I developed a method which permitted me to adjust the filter elements and temperature so that not only was the band-pass 0.5 angstroms, but it was positioned within 0.1 angstrom of the 6562.85 angstroms. Furthermore, the energy in the red 0.25-angstrom side was within 2% of the energy in the 0.25-angstrom blue side. In other words, the transmission pattern was centered on 6562.85 angstroms to within 0.1 angstroms and it was 98% symmetrical to both sides of this center wavelength.
This had never been done and several world-class solar astronomers discovered that they had not been seeing and photographing the wavelength they thought. They certainly didn't publish anything announcing this but they virtually stood at my spectroscopy lab door waiting for me to calibrate their filters -- the Air Force did the same.
At that time, I was in the Optics Section, Astronomy Branch, Space Physics Division, and we were doing a lot of optical design and fabrication in our own optics laboratory. Most optical tests were done relative to the wavelength of some monochromatic light.
Optics ground by the hand of amateur astronomers in their back yards can be made true to within the distance of 1/4-wave of light. Cheapie commercial telescopes could be within 1/8-wave -- but what 1/8-wave of what color light?
We would not want to use red light -- these are the longer wavelengths. Since mercury vapor lamps are readily available and being toward the blue end of the spectrum (shorter wavelengths), that is a popular reference. Mercury's wavelength is 3838 angstroms, or 0.000015". Therefore, at an 1/8-wave of mercury, the lenses of a cheapie telescope could be true to 0.000002".
I remind you now of the idiot statement by the administrator of Neo-NASA, who, while explaining the "minor" difficulties with Hubble, said that it was "only off by the width of a human hair."
The width of a human hair is 0.002"!
So if Hubble had only 1/8-wave design limits (0.000002") then according to the highest office in NASA, Hubble was off by 0.002" (human hair).
Or worded another way; Hubble was out of focus by 1,000 times what could be expected from Wal-Mart's El Cheapo, Christmas Special telescopes.
Some smart person once said, "There will no longer be minor errors." (Oh, yeah. I forgot...I said that.)
The next two images will involve images of the sun -- sort of.
Ken Cashion
Next Long & Short Image du Jour